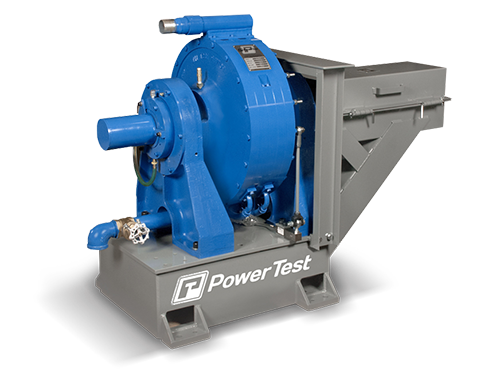
We Make Your Testing Easy
Dynamometers and Heavy Equipment Testing Systems

Engine Dynamometers
Engine Dynamometer testing solutions for your equipment, ranging from 20-10,000 horse power.

Transmission & Hydraulic Test Stands
For complete verification of all of your transmissions, pumps, and other hydraulic components.

Chassis Dynamometers
Eliminate costly test drives and provide documented proof of quality for truck and bus repairs.

Custom Engineered Solutions
Industry-leading expertise for testing solutions in powertrain, rotating equipment, and fluid power industries.
An Industry Leader in Dynamometers and Heavy Equipment Testing Systems
Power Test, LLC is an industry leader in the design, manufacture, and sale of dynamometers, heavy equipment testing systems and related data acquisition and control systems. For over 40 years, Power Test has provided specialized test equipment to manufacturers, rebuild facilities, and distributors in the mining, oil & gas, power generation, marine, trucking, construction, rail, and military markets in over 90 countries on six continents. Our headquarters and manufacturing operations are located in Sussex, WI with sales representatives worldwide.
The Power Test team of innovative engineers, designers, software developers, and sales consultants will SOLVE YOUR CHALLENGES with logical solutions. Our skilled machinists, fabricators, electronic technicians, and assemblers build products to meet your unique needs. Our technical service experts are dedicated to working with you, anywhere and anytime. They travel the globe to ensure your equipment is running right and your staff is trained to operate it. Our exceptional product life and manufacturing expertise make us an industry-leading dynamometer manufacturer, as evidenced by our first machine sold, which remained operational for 42 years.